Page 98 - Šolsko polje, XXIX, 2018, št. 3-4: K paradigmam raziskovanja vzgoje in izobraževanja, ur. Valerija Vendramin
P. 98
šolsko polje, letnik xxix, številka 3–4
basic arithmetic truths, there is an arithmetical statement that is true, but not
provable in the theory.
This theorem was designed to prove inherent limitations (incom-
pleteness) for axiomatic systems for mathematics, but what Cohen and
Nagel are claiming is, mutatis mutandis, an application of Gödel’s (first)
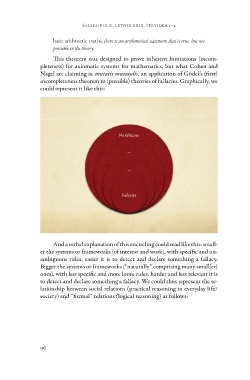
incompleteness theorem to (possible) theories of fallacies. Graphically, we
could represent it like this:
And a verbal explanation of this encircling could read like this: small-
er the systems or frameworks (of interest and work), with specific and un-
ambiguous rules, easier it is to detect and declare something a fallacy.
Bigger the systems or frameworks (“naturally” comprising many small(er)
ones), with less specific and more loose rules, harder and less relevant it is
to detect and declare something a fallacy. We could thus represent the re-
lationship between social relations (practical reasoning in everyday life/
society) and “formal” relations (logical reasoning) as follows:
96
basic arithmetic truths, there is an arithmetical statement that is true, but not
provable in the theory.
This theorem was designed to prove inherent limitations (incom-
pleteness) for axiomatic systems for mathematics, but what Cohen and
Nagel are claiming is, mutatis mutandis, an application of Gödel’s (first)
incompleteness theorem to (possible) theories of fallacies. Graphically, we
could represent it like this:
And a verbal explanation of this encircling could read like this: small-
er the systems or frameworks (of interest and work), with specific and un-
ambiguous rules, easier it is to detect and declare something a fallacy.
Bigger the systems or frameworks (“naturally” comprising many small(er)
ones), with less specific and more loose rules, harder and less relevant it is
to detect and declare something a fallacy. We could thus represent the re-
lationship between social relations (practical reasoning in everyday life/
society) and “formal” relations (logical reasoning) as follows:
96